My Homepage
This page is part of the
Mozilla Open Directory
project
Sample Card (fattened)

Ccard 2.0

or: How to make fun out of something highly abstract.

Ccard is a card game. You can download the cards as gzipped postscript It was born in an area of distress in May 1999, kicked of by the Summer School in Semantics (at BRICS, Aarhus University, Denmark) and in particular the course about category theory there.

How to play?

There are some simple "rules" I made up for two or more players (but you are of course free to change them).
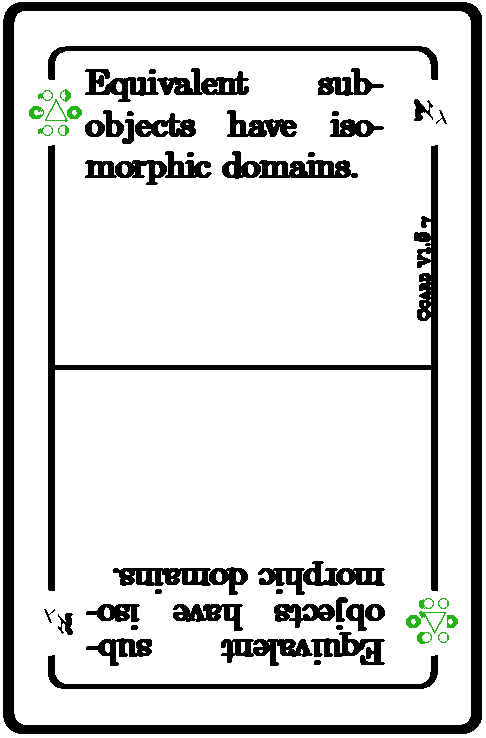
  1. The seven suits are organized by a increasing number of "circles" which are meant to reflect the "difficulty" of the facts within. The number of circles/triangles of the suite symbol determines the rank of this suite.
  2. Every suite has nine cards. The highest card of one suit is the "aleph"_lambda (resembles a shaky N), followed by "omega", "infinity", then 11, 7, 5, 3, 2 (I like to stick with prime numbers) and finally the empty set (or "naught").
  3. Each of 2 (or possibly more) players gets six cards, the rest is left as a pile on the table.
  4. The game proceeds in rounds. Every round is played counterclockwise, where every player plays one card.
  5. The player winning a round gets all the cards played there. The player who won the most rounds, wins the game.
  6. The highest card played wins the round. This is either the one with the highest suite (i.e. the maximal number of "circles") or the highest card within the highest suite played, if there is a conflict.
  7. If a player tries to "take over" by playing a so-far highest card, but without increasing the suite (!) he has to explain the fact written on the card. If he cannot do that or the other players agree the explanation was unsatisfactory, his card has no effect.
Note that rule 7. is the only one where the text on the cards has some relevance (other than just having it in front of your eyes). It might take an experienced player to trump a card in e.g. a 4-circle-suite. It is recommended not to play it in public areas where there are people around you might consider one day to ask out for a date.

What is category theory?

The ugly truth is: I don't know. And I was not able to spot someone who as well knew it and was able to explain it to me (the more you are affiliated with category theory the more you seem to lose explanatory skills). But the comments describing it best were in the direction "A methodology of organizing mathematics" (Jaab van Oosten). There are mathematicians like McLarty claiming it is also a foundation of mathematics, but I am not the only one doubting that.

Why should one study it?

Coming from the computer science side, I'm of the opinion that mathematical structures are not itself a good reason to study them - I'd like to _use_ them, to express (and exploit) properties and prove theorems that enable me actually to _do_ things (rather than "being able to in principle"). So why is it useful? The answers I found range from philosophical to pragmatical; at first it provides an expressive notation in a reasonable abstract to savior one from all the gory details. This makes it easier (or even at first possible) to spot identities and similarities of definitions. At second, it provides a short and elegant proof technique - especially the representation of primitive recursion via the natural numbers object (e.g. McLarty's book) and the solution of recursive domain equations without using the by-foot information-system construction (e.g. Pierce) caught my attention. Finally I believe an abstract view can be very valuable in understanding a problem in the occasions one has to do that in depth. To quote Adámek et. al,p.4 : "(It) will help those who are confronted with a new field to detect analogies and connections to familiar fields (...) Categorical knowledge thus helps to direct and to organize one's thoughts." It might be a good idea, not to think to hard about foundation issues.

Good books?

I haven't found any. That does not mean that there are no books at all, but every single I encountered lacked some essential contents. What I'd like to recommend as an introduction is a combined reading of Sibylle Fröschle pointed me later to another quite comprehensive book I feel inclined to add to this list: There are also some BRICS lecture notes by Jaap van Oosten ("Category Theory in Computer Science", BRICS lecture series BRICS-LS-95-1 (Issue May 1999)) hopefully soon available at http://www.brics.dk/LS/Abs/BRICS-LS-Abs/BRICS-LS-Abs.html#BRICS-LS-95-1 However, it is difficult to read and contains more examples and definitions than explanations. If you want to use it nevertheless, you might find this rough INDEX helpful.

In October 2001, Lion Kimbro suggested the book Lion describes it as "very nicely explained, nice problems, visual explanations". I only took a brief look at it, and based on that I tend to agree. The book evolved from a lecture course that was taught repeatedly.

So what is this strange Ccard all about?

Ccard is a card "game" or rather a memory trick. When studying category theory I realized that there was an abundance of facts that one has to keep in mind in order to understand the next step. At first these occurred mysterious to me (I called them "mantras" in a good old AI tradition, for they reflected some deeper truth that was not so easy to grasp). I found it helped to keep them in sight, e.g. by laying a patience with these cards from time to time. By the way - the "C" in "Ccard" is meant to resemble the letter usually associated with "category"... so "Ccard" is something quite nerdy, but you might like the thought of just playing with highly abstract math.

Why this second version?

I have to admit that version 1.0 has various weaknesses, especially in the selection of the card texts. After some time I found them too unrelated and the suites did not seem to be too coherent in their contents.
In version 2.0 this is hopefully fixed to a vast extend. Also, a sixth and seventh suite were added (coming closer to the ultimate goal of reaching the magic number of nine). It is still up to discussion, so I prefer to call it the "gamma" version. Version 1.0 is still available (though not recommended): In a way, this game is still work in progress (as learning category theory is rather a journey than a goal). So I am grateful for any suggestions or feedback.

Where do the texts on the cards come from?

I copied them from lecture notes and books. A complete reference is available (where e.g. BP35: (...) is to be interpreted as: This fact is found in Benjamin Pierce's book, page 35).
I was quite thorough with my retrieval of them, however I cannot guarantee that they are 100% error free or lacking some important context in order to make them meaningful (remember, I was myself in the stage of learning this when I designed it). Moreover, since category theorists -- like all mathematicians with some self respect -- seem to be unable to agree on one notation, I also had to smooth this out. So if you should spot peculiarities, please do not hesitate to contact me: omoeller@verify-it.de.

Have fun with this abstract nonsense. I hope you enjoy it.

Note: Apparently putting this game into the Mozilla pages, made marketing industry alert of its existence. Here is a MAIL I received on 17 August 2001.

Last words: I'd like to thank all the people at BRICS I annoyed while adopting some basic knowledge about category theory, especially Paola and Riko, that they still are willing to talk to me and did not take me to a mental home.
I reckon it must have been tempting more than once.

counter started on 23/12/2001 counter started on 23/12/2001

webmaster - Last modified: Wed Jul 31 12:34:39 2002